Imagine a world where every geometric shape holds a hidden treasure – its volume. This intriguing concept, the measurement of the space a three-dimensional object occupies, becomes particularly fascinating when we delve into the realm of prisms and cylinders. In this exploration, we’ll embark on a journey to demystify the intricacies of calculating the volumes of these shapes, specifically focusing on the “12-4” rule – a key principle that simplifies these calculations.
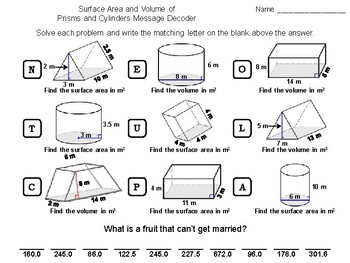
Image: www.teacherspayteachers.com
Understanding volume is not just an academic exercise. It’s a cornerstone of real-world applications, from determining the capacity of a container to designing efficient structures. Mastering the techniques to calculate volumes of prisms and cylinders empowers you to tackle problems in architecture, engineering, and even everyday life.
Exploring the Fundamentals: Prisms and Cylinders
Before we dive into the “12-4” rule, let’s establish a strong foundation by defining our geometric stars: prisms and cylinders.
Prisms: A World of Straight Edges
Imagine building a 3D shape by stacking identical polygons, one on top of the other. The result? A prism! Prisms have two congruent, parallel bases connected by rectangular faces. They come in various shapes depending on their base, like triangular prisms, rectangular prisms, or even hexagonal prisms.
Think of a building block, an ordinary box, or a slice of cheese – all these everyday objects can be classified as prisms.
Cylinders: Rolling with a Radius
Next up, we have cylinders. Picture a circular base, and then a curved surface that smoothly connects it to a parallel, congruent circular base. That’s a cylinder! Think of a can, a pipe, or even a roll of toilet paper – these familiar objects exemplify cylinders.
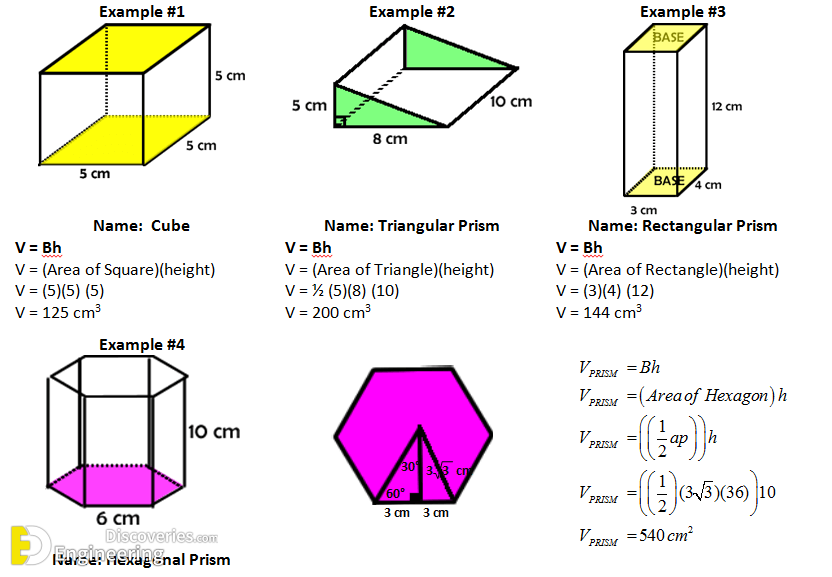
Image: engineeringdiscoveries.com
Unveiling the “12-4” Rule: A Shortcut to Volume
Now, let’s unveil the secret behind the “12-4” rule, a powerful tool for calculating volumes of both prisms and cylinders.
The “12-4” rule essentially provides a shortcut for finding the volume of a prism or cylinder by employing this simple formula:
Volume = (1/2) * (12 – 4 * number of sides) * area of base * height
It’s important to note that this rule applies specifically to prisms and cylinders where the bases have a regular polygon shape – a polygon where all sides and angles are equal. For irregular base shapes, we’ll need a more general formula.
Unpacking the “12-4” Rule: A Step-by-Step Guide
Let’s break down how to apply the “12-4” rule through an illustrative example. Suppose we have a triangular prism. We will follow these steps:
1. Identifying the Number of Sides
A triangle has three sides.
2. Plugging into the Formula
Our formula becomes:
Volume = (1/2) * (12 – 4 * 3) * area of base * height
3. Calculating the Area of the Base
We’ll need to know the specific dimensions of the triangular base to calculate its area. For example, if the base has a base length of 5 cm and a height of 4 cm, the area would be (1/2) * 5 cm * 4 cm = 10 cm².
4. Determining the Height
The height is the distance between the two bases. If the height of the prism is 7 cm, we’re ready for the final step.
5. Putting it Together
Now, we can plug in all the values:
Volume = (1/2) * (12 – 4 * 3) * 10 cm² * 7 cm
Volume = (1/2) * (0) * 10 cm² * 7 cm
Volume = 0 cm³
Beyond “12-4”: General Volume Formulas
While the “12-4” rule is a convenient shortcut for regular polygons, we need more versatile formulas for general prisms and cylinders. Let’s explore those now:
General Formula for Prisms
The volume of any prism is simply the area of its base multiplied by its height.
Volume = Area of base * height
General Formula for Cylinders
The volume of a cylinder is the area of its circular base multiplied by its height.
Volume = πr² * height
where:
π ≈ 3.14159 (a mathematical constant)
r = radius of the base
height = distance between the bases.
Real-World Applications: Where Volume Takes Center Stage
Now that we’ve explored the fundamentals, let’s see how these volume concepts play out in real-life scenarios.
1. Filling Up Your Tank
Imagine you’re driving your car and need to fill up your tank. The gas tank is essentially a cylinder. To determine how much gasoline it can hold, you’d use the cylinder’s volume formula.
2. Constructing a Giant Pool
Suppose you’re building a rectangular pool in your backyard. To calculate how much water it can hold, you’d use the prism’s volume formula. This would help you determine the amount of water needed to fill it up.
3. Packaging a Gift
When wrapping a gift, you might wonder how much wrapping paper you’ll need. This boils down to calculating the surface area of the gift box. Knowing the box’s volume can help you estimate the amount of wrapping paper required.
12-4 Volumes Of Prisms And Cylinders
Final Thoughts: From Geometry to Reality
The journey into the world of prisms and cylinders has been an exploration of geometry’s practical applications. By mastering the “12-4” rule and general volume formulas, we gain the power to calculate the volumes of countless shapes, solving real-world challenges in various fields. Remember, this is just the beginning. As you delve deeper into the realm of geometry, you’ll unravel even more fascinating concepts and discover how math plays a vital role in shaping our world.




