Imagine you’re building a complex puzzle, each piece representing a term in an algebraic expression. Multiplying a polynomial by a monomial is like fitting those puzzle pieces together, creating a new, larger structure. But how do you master this skill and confidently navigate the world of algebraic expressions? Let’s unlock the secrets behind this fundamental concept, starting with the basics and exploring its real-world applications.

Image: www.pdfprof.com
Understanding the terminology is your first step. A monomial is a single term, like 3x or 5y². A polynomial is a combination of multiple terms connected by addition or subtraction, such as 2x² + 5x – 1. Multiplying a monomial by a polynomial involves distributing the monomial across all the terms in the polynomial. This simple rule sets the foundation for manipulating complex algebraic expressions and solving a wide array of problems in various fields like physics, engineering, and finance.
Breaking Down the Multiplication Process
Multiplying a monomial by a polynomial is like applying a scaling factor to each piece of the polynomial. Think of it like enlarging a photograph – each part of the picture gets proportionally bigger. Let’s break it down step by step:
1. Distributive Property: The Key to Success
The distributive property is the cornerstone of multiplying a monomial by a polynomial. It states that multiplying a sum by a number is the same as multiplying each term of the sum by the number individually. Remember that a polynomial is essentially a sum of terms. Thus, when you multiply a monomial by a polynomial, you distribute the monomial to each term within the polynomial.
2. Multiplying Coefficients and Variables
Once you’ve distributed the monomial, you multiply the coefficients (the numerical parts) and the variables. Remember, when multiplying variables with exponents, you add their exponents. This is how the power of the variable changes in the final product.
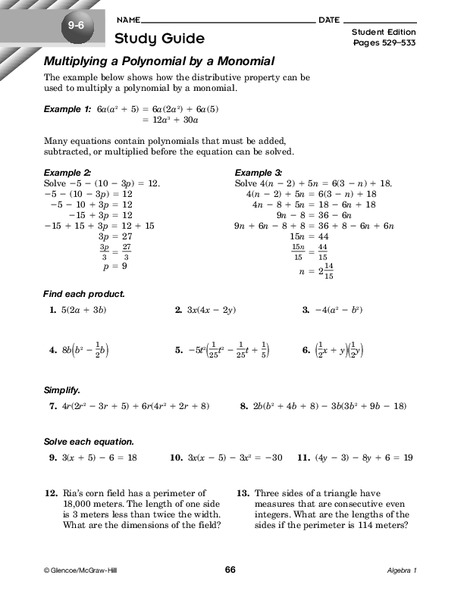
Image: www.lessonplanet.com
3. Combining Like Terms: Simplifying the Result
After multiplying each term, you may find like terms in your expression. Like terms are terms with the same variables raised to the same powers; they can be combined by adding their coefficients. Simplifying the expression by combining like terms ensures a concise and clear representation of your answer.
Real-World Applications
Multiplying polynomials by monomials is not just an abstract mathematical exercise. It finds real-world applications in various disciplines.
1. Engineering and Physics:
In engineering, polynomials are often used to model the behavior of physical systems, such as the motion of a projectile or the flow of electricity. Multiplying a polynomial by a monomial can adjust these models, incorporating various factors like changes in mass, velocity, or resistance.
2. Finance:
Polynomials can also be used to represent financial data, such as the growth of an investment portfolio over time. Multiplying by a monomial can help adjust these financial models to account for different interest rates, inflation, or other market variables.
3. Computer Programming:
In computer programming, polynomials are used in various algorithms, including those for image processing and data analysis. Multiplying a monomial by a polynomial can be used to manipulate data efficiently, allowing for faster processing and more accurate results.
Examples to Illuminate the Concept
Let’s look at some examples to solidify your understanding of the process:
Example 1: Simple Multiplication
- Multiply 2x by (3x² + 4x – 5)
Applying the distributive property:
(2x)(3x²) + (2x)(4x) + (2x)(-5)
Multiplying the coefficients and variables:
6x³ + 8x² – 10x
The final result is the polynomial 6x³ + 8x² – 10x.
Example 2: Working with Exponents
- Multiply 3y² by (2y³ – 4y² + 5y)
Applying the distributive property:
(3y²)(2y³) + (3y²)(-4y²) + (3y²)(5y)
Multiplying the coefficients and variables (remember to add exponents):
6y⁵ – 12y⁴ + 15y³
The final result is the polynomial 6y⁵ – 12y⁴ + 15y³.
Example 3: Combining Like Terms
- Multiply -5a by (2a² – 3a + 1)
Applying the distributive property:
(-5a)(2a²) + (-5a)(-3a) + (-5a)(1)
Multiplying the coefficients and variables:
-10a³ + 15a² – 5a
The final result is the polynomial -10a³ + 15a² – 5a.
Practice Makes Perfect
The more you practice multiplying monomials by polynomials, the more comfortable you will become with the process. Start with simple examples and gradually work your way up to more complex problems. There are many online resources, including interactive exercises and quizzes, available to help you practice and hone your skills.
8 2 Practice Multiplying A Polynomial By A Monomial
Conclusion
Mastering the art of multiplying a monomial by a polynomial is essential for navigating the world of algebra and its applications. It’s a key building block for working with higher-order expressions and tackling complex mathematical problems across various disciplines. By understanding the distributive property, multiplying coefficients and variables, and combining like terms, you can confidently navigate this powerful mathematical tool. So, embrace the challenge, practice consistently, and watch as your understanding blossoms, turning the intricate world of polynomials into a manageable and rewarding journey.




